Hardest SAT II Math Question Ever
- Joel Runevic

- Sep 4, 2020
- 3 min read
If you are not a member of OnlyPhysics, please consider joining us - it's completely free and comes with loads of benefits, such as free online tutoring.
Welcome to a relatively short post on arguably the most challenging SAT II Math Question ever. If you aren’t aware, the SAT II is a 50 Question test that High Schoolers sit as part of their application process to US Universities. However, this question is useful for any High School Math Student, regardless of their nationality. Let’s have a look at the question then shall we?
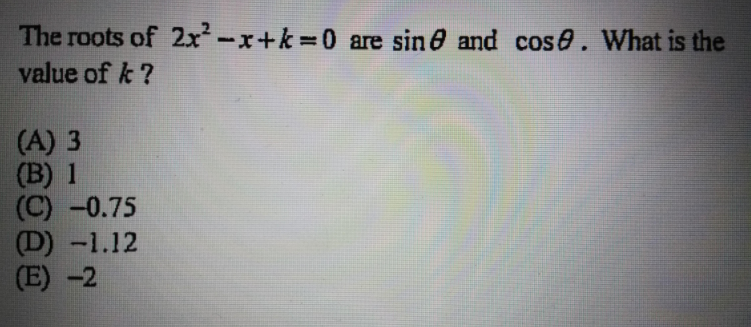
Now, this is a multiple choice question and a calculator is allowed in the SAT II. Thus, we are only interested in obtaining the answer by the quickest means possible. Typically, one should only allocate about a minute to do this question.
Your first inkling may have been to sub in sin(θ) and cos(θ) into the above equation to form two different equations. From here, we can equate the two equations together, eliminate k, and solve for θ. This will then allow us to sub back our value for θ into the original equation, allowing us to calculate the value for k. Perfect, right?
Well, unfortunately not. You see this would work if we didn’t run into this horrible equation right here:
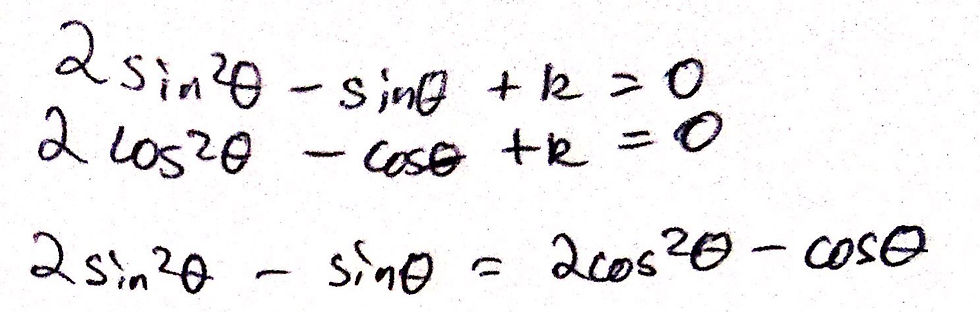
Solving this is a nightmare. I mean we can easily express sin^2(θ) in terms of cos(θ), by using our trigonometric identity sin^2(θ) + cos^2(θ) = 1. However, to express sin(θ) in terms of just cos(θ) is impossible. Therefore, we must think of a different way to solve this problem.
We can do so by using our trusty tool - the quadratic formula. This is quite uncommon for High School Maths, such as UK Year 12-13, as typically you use the quadratic formula in the lower years. However, as you know, the quadratic formula can be used to calculate the roots of a quadratic polynomial. The equation is as follows:
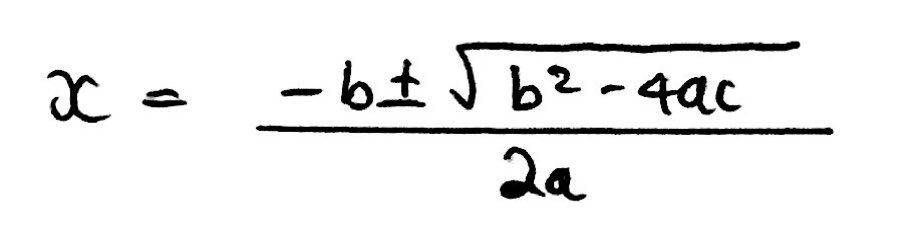
Many, however, don’t question where such an equation comes from. Despite being beyond the typical curriculum, it is really useful to understand the derivation of the quadratic formula as it uses a skill that is very much tested in High School Maths - Completing the Square.
If you still aren’t interested, then scroll down until you reach the section titled ‘The Clever Little Trick’. Otherwise, continue reading.
Deriving the Quadratic Formula
We know the general expression for a quadratic polynomial is as follows:

Thus, we can complete the square on this general equation in order to form a general formula that we can use to calculate the roots of a polynomial.
Completing the square, as you may remember, is just an alternative method to calculate the roots of a quadratic if you are struggling to factorise the polynomial. It also finds you the turning point of the function; however, it is much better to just use differentiation for this purpose.
Before we begin to complete the square on the polynomial, we must factor out both sides by the x^2 coefficient as shown below. This is what you must do whenever a > 1.

From here, we can now complete the square as follows. Be careful not to make silly mistakes as it can get quite confusing with all the a’s, b’s and c’s floating around. Just follow your basic algebraic manipulation rules and you should be fine!
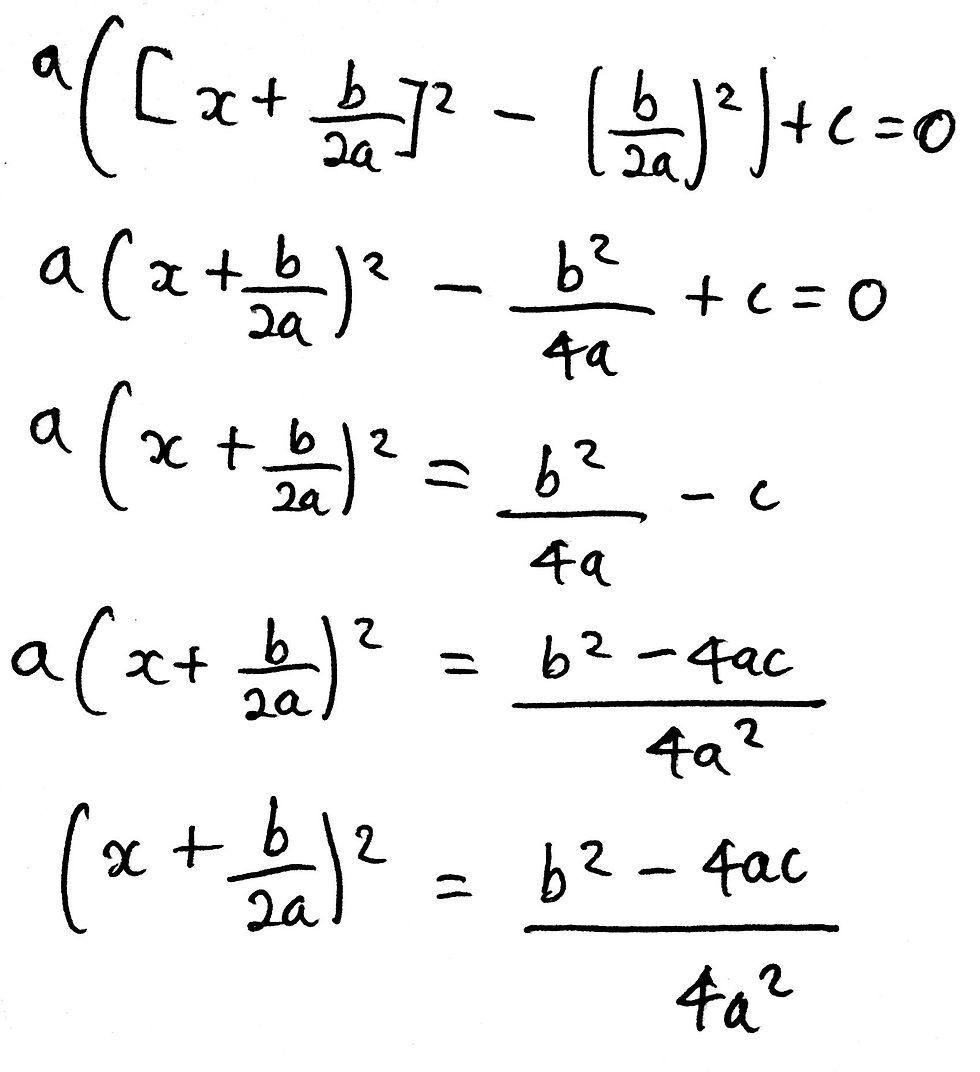
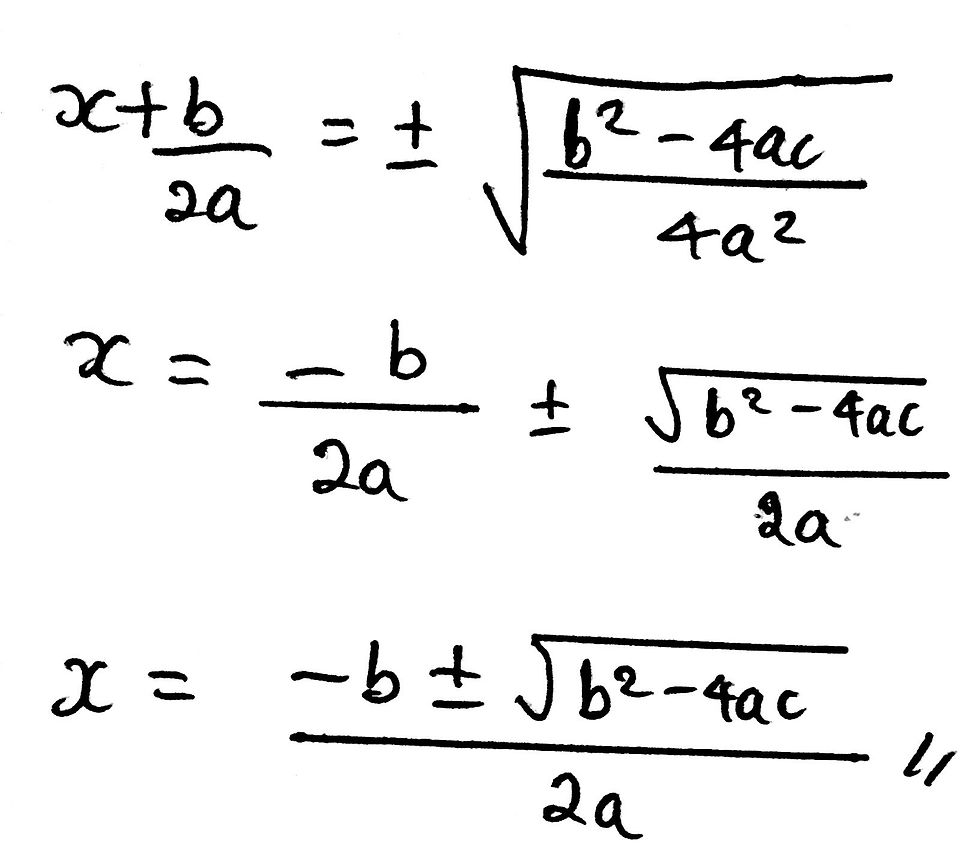
The Clever Little Trick
Now that we have derived the Quadratic Formula and thus have a much greater insight into how the formula works, we can begin using it to solve this question. We know the values of a, b and c; a is 2, b is -1, and c is k. We know that the roots are sin(θ) and cos(θ) respectively. Thus, we can sub in our values of a, b, and c into the quadratic formula and obtain relevant equations for sin(θ) and cos(θ) respectively.
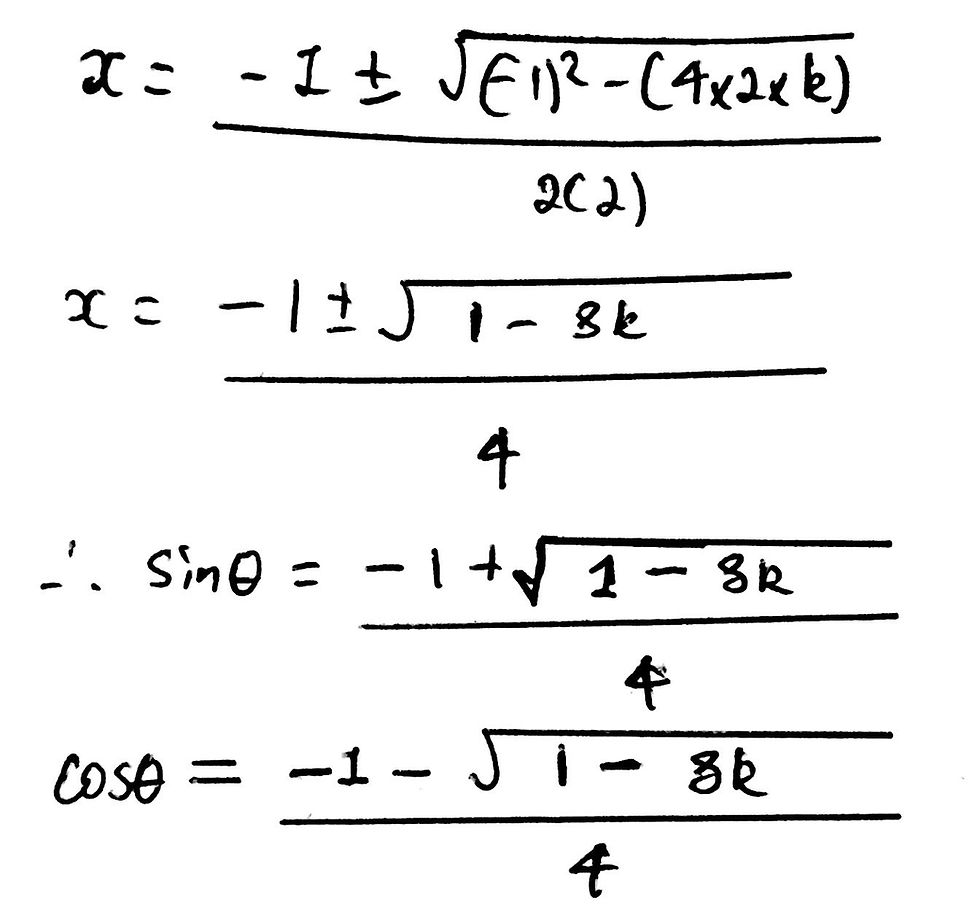
We can now use a well-known trigonometric identity: sin(θ)^2 + cos(θ)^2 = 1 as we have equations for sin(θ) and cos(θ) respectively. We can now therefore solve for k. I have included my workings below.

That is it for this post. This question, though a short question, certainly has a lot of potential to teach High School students a lot of concepts. Moreover, it is a SAT Math II question and thus has the potential to come up in future tests, if you are planning to sit the test.
Thank you very much for reading this post! I hope that it has been helpful to you. If you have any questions, post them down in the comments below. Stay tuned for future posts!



Comments